Two particles of masses my and m, have equal kinetic energies. The ratio of their momenta is (A) mm2 (B) m2: m (C) m, : m2 (D) m:m 10 The nessure the
Por um escritor misterioso
Last updated 21 fevereiro 2025
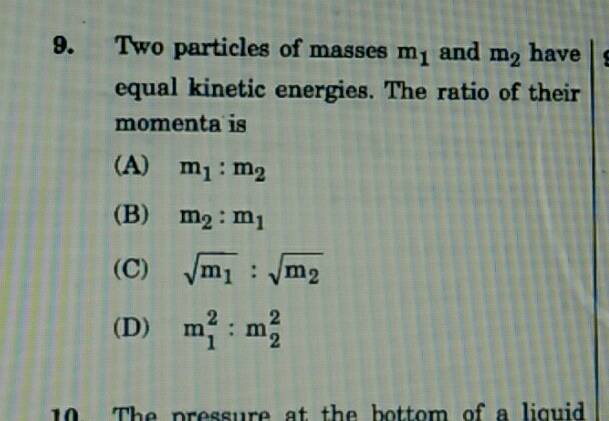
Click here:point_up_2:to get an answer to your question :writing_hand:two particles of masses my and m haveequal kinetic energies the ratio of theirmomenta isa
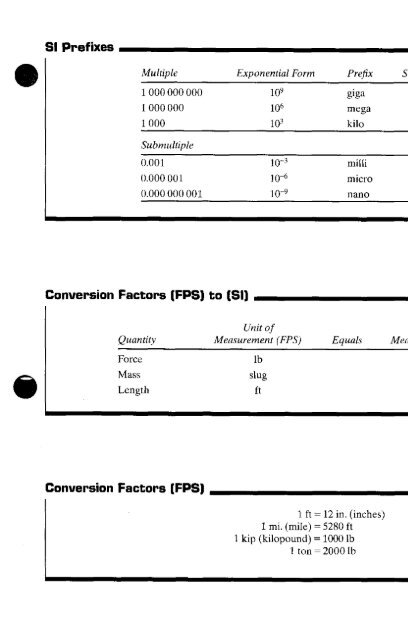
Engineering.Mechanics.STATICS.Fourteenth.Edition
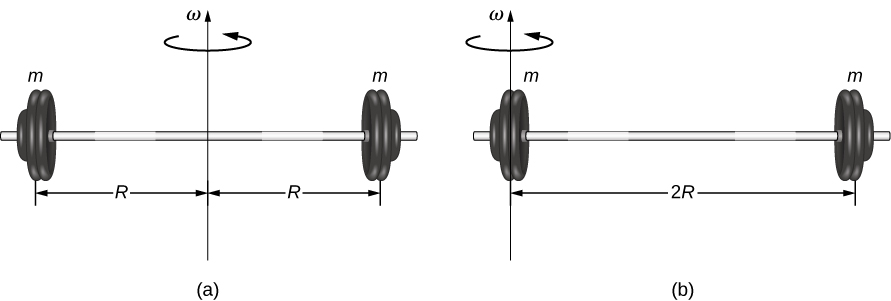
10.5 Calculating Moments of Inertia
Two particles of masses m and 2m are revolving in circular orbits due to mutual gravitational force of attraction. Then ratio of their kinetic energy is

Two masses m, = 1 kg and m, = 2 kg are connected by a light inextensible string and suspended by means of a weightless pulley as shown in the figure. Assuming

10.5 Calculating Moments of Inertia

25. Two particles with equal kinetic energies are having masses in the ratio of 1 : 2. Then linear momenta will be in the ratio- (1) 1 (2) 4 (3) 0.707 107 (4) 2
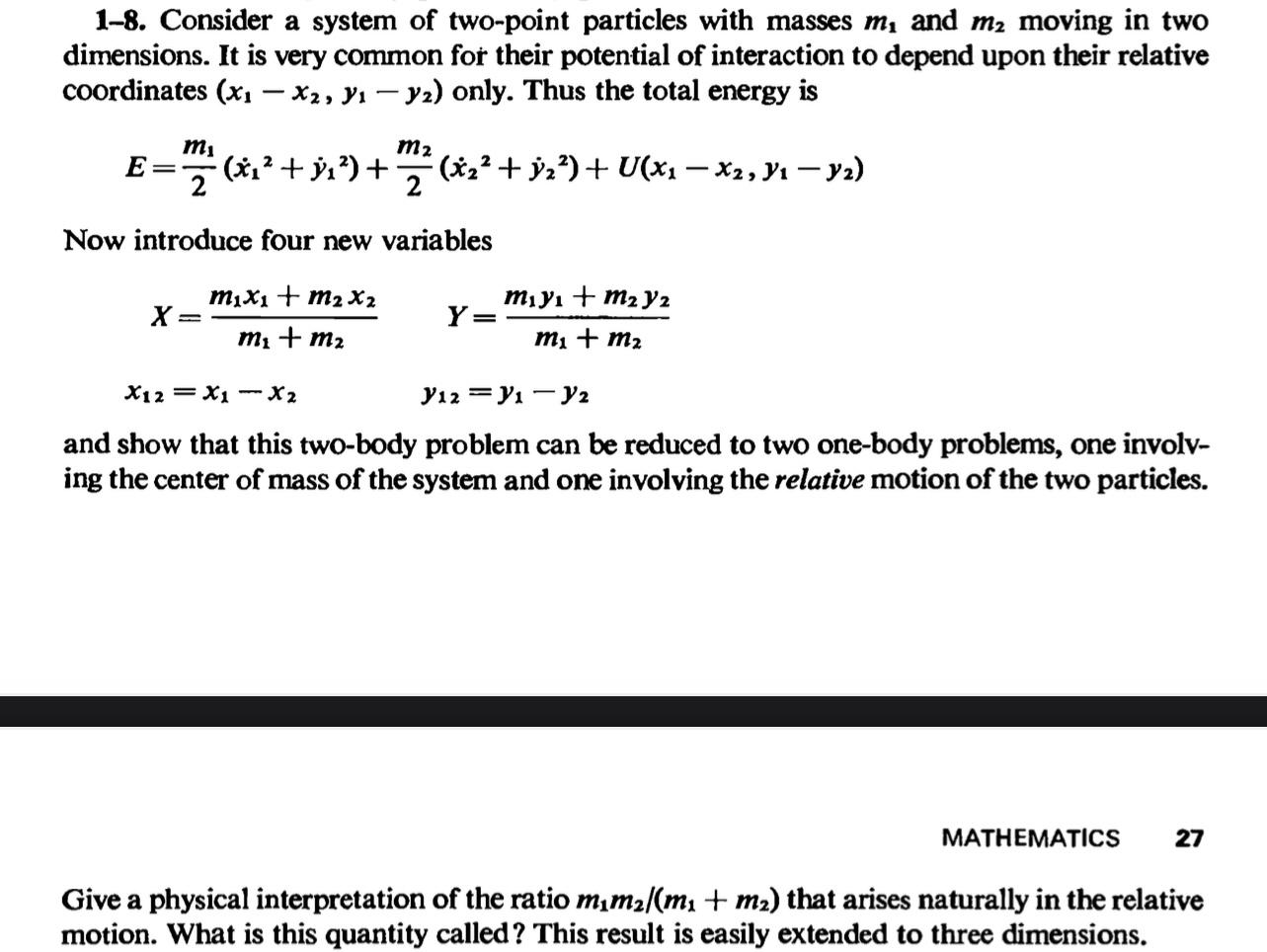
Solved 1-8. Consider a system of two-point particles with

volume-2 by chandan kumar - Issuu

TUTORIAL No. 1 FLUID FLOW THEORY In order to complete this
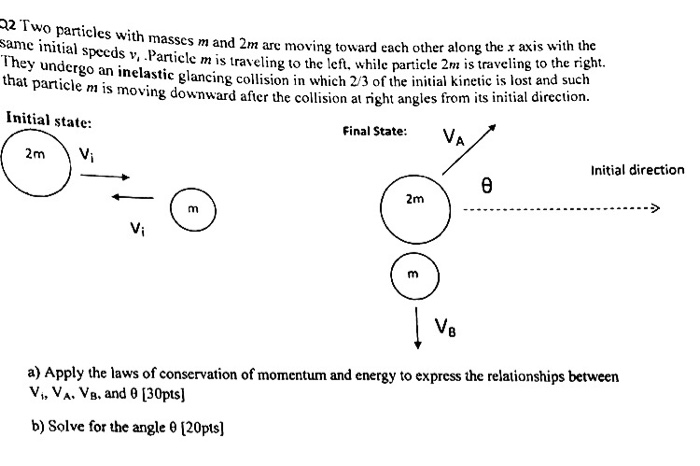
Solved Two particles with masses m and 2m are moving toward
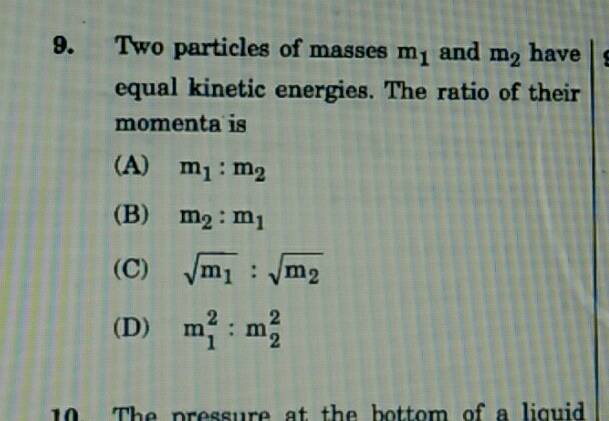
Two particles of masses my and m, have equal kinetic energies. The ratio of their momenta is (A) mm2 (B) m2: m (C) m, : m2 (D) m:m 10 The nessure the
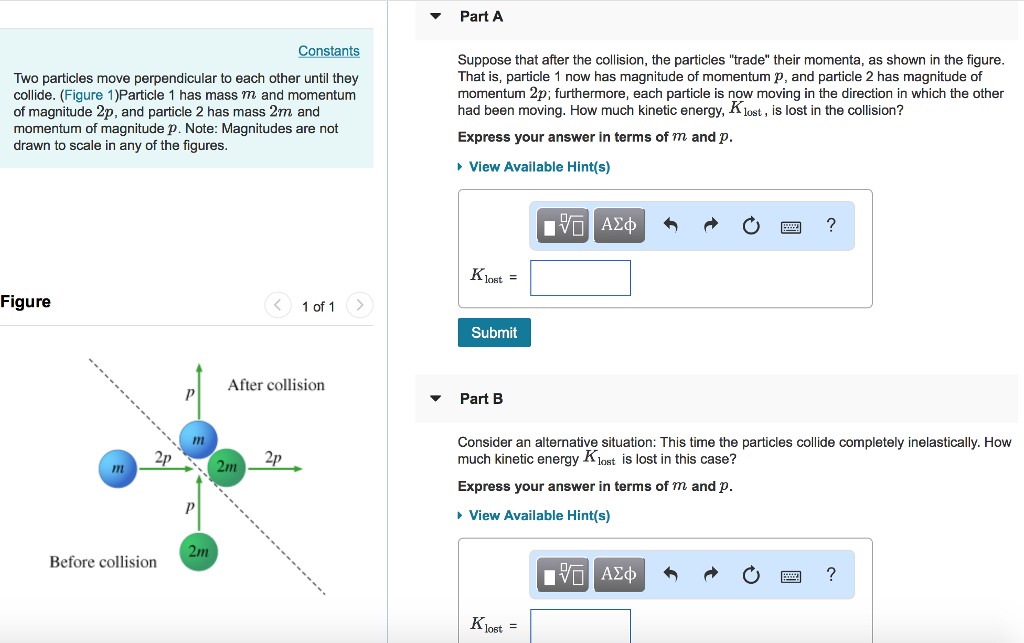
Solved VPart A Constants Two particles move perpendicular to
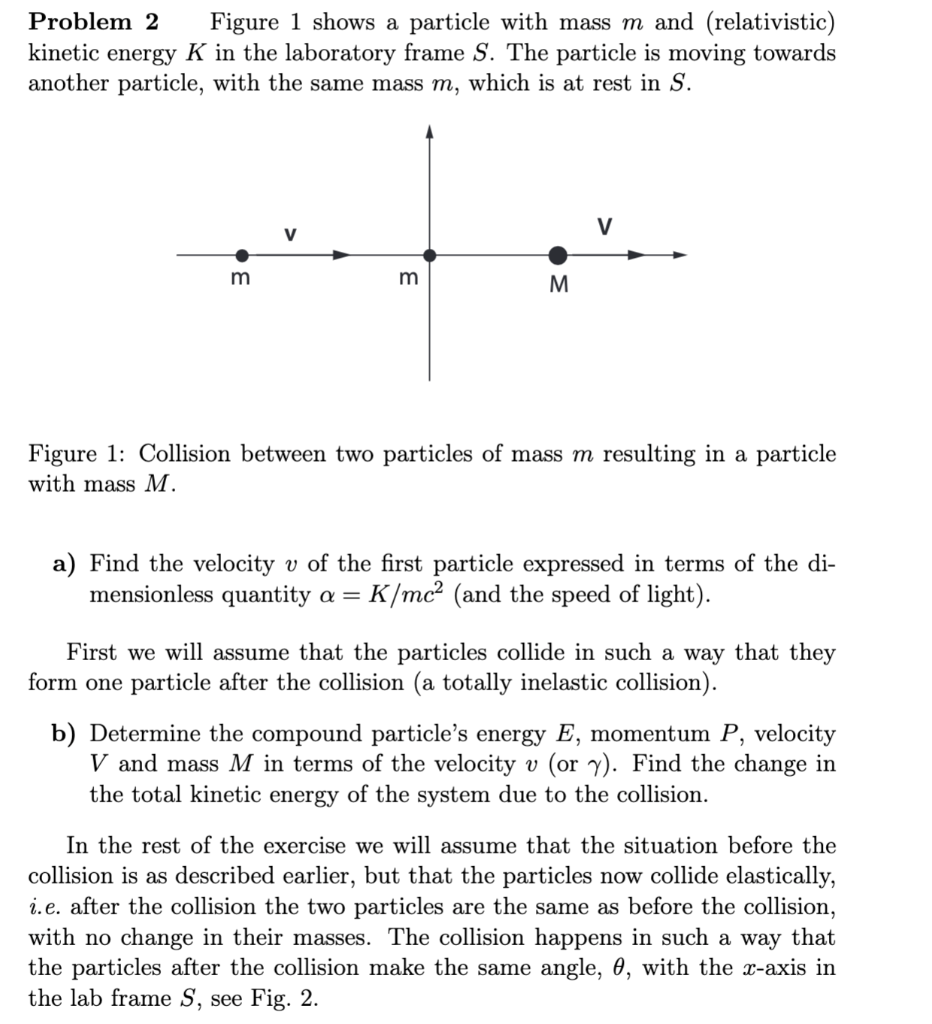
Solved Problem 2 Figure 1 shows a particle with mass m and
Recomendado para você
-
 Conversión De G/mm2 A G/m2 Conversión De G/mm2 A G/m2 By, 44% OFF21 fevereiro 2025
Conversión De G/mm2 A G/m2 Conversión De G/mm2 A G/m2 By, 44% OFF21 fevereiro 2025 -
 Sterilization box for M2 heads and rings - Moria - Ophthalmic Instruments21 fevereiro 2025
Sterilization box for M2 heads and rings - Moria - Ophthalmic Instruments21 fevereiro 2025 -
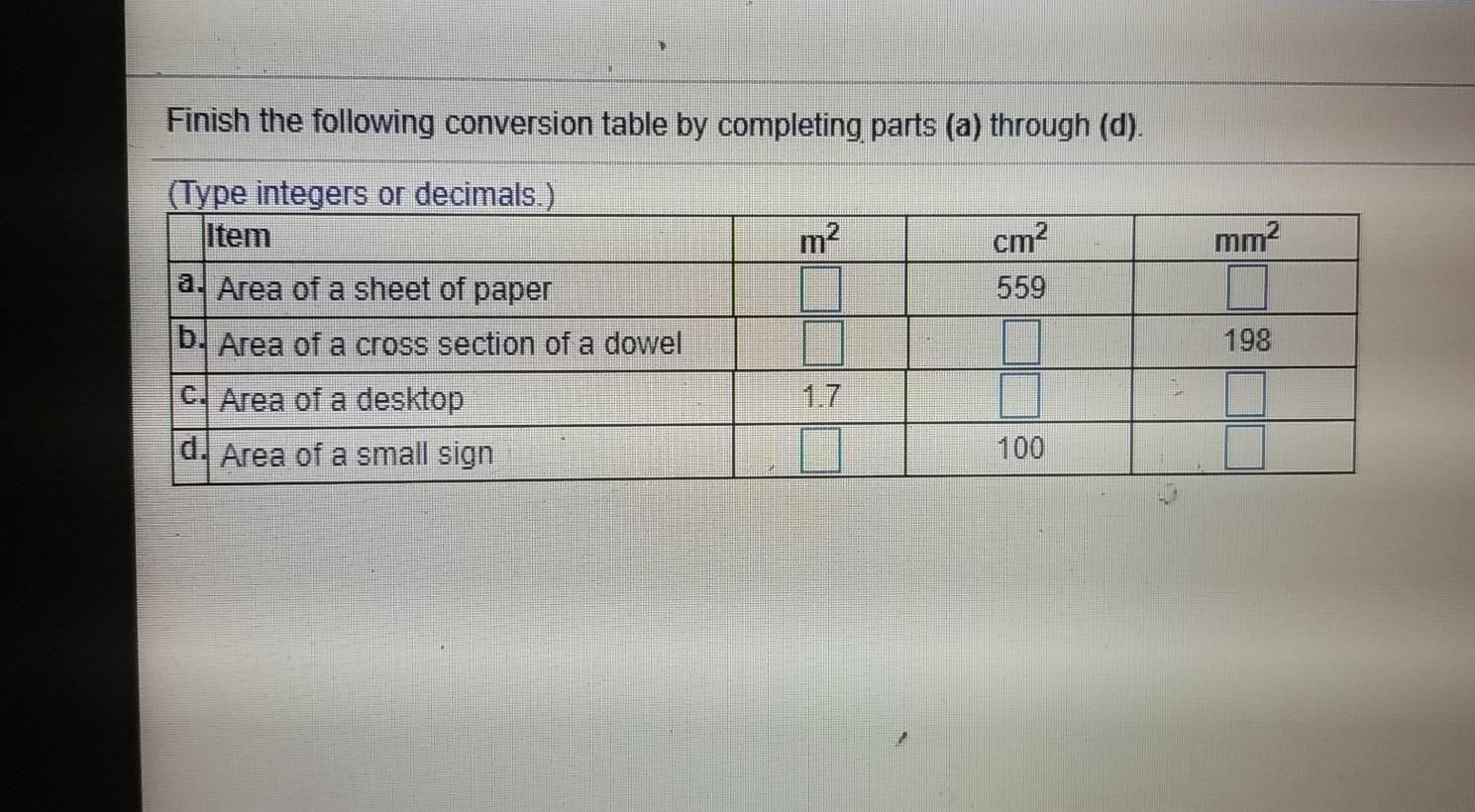
Solved Finish the following conversion table by completing21 fevereiro 2025
-
 N/m2 to N/mm221 fevereiro 2025
N/m2 to N/mm221 fevereiro 2025 -
 AIRIC Heat Shrink Wire Connectors 100pcs Marine Crimp Terminals 22-10 Gauge Butt Splice Kit21 fevereiro 2025
AIRIC Heat Shrink Wire Connectors 100pcs Marine Crimp Terminals 22-10 Gauge Butt Splice Kit21 fevereiro 2025 -
 Chuyển đổi Milimet vuông (mm2) sang Mét vuông (m2)21 fevereiro 2025
Chuyển đổi Milimet vuông (mm2) sang Mét vuông (m2)21 fevereiro 2025 -
 SOLVED: please solve all parts of question 2. thanks! 2. Compute the and optimal strategies given the following: Pm=W1,W2,W3,W4 Pw1=m2,m3,m1,m4,ms Pm=W4,W2,W3,W1 P(w=m3,m1,m2,m4,m5 Pm3=W4,W3,W1,W2 Pw=m5,m4,mm2,m3 Pm4=W1,W4,W3,W2 Pw4=m1,m4,m5,m2,m3 Pms21 fevereiro 2025
SOLVED: please solve all parts of question 2. thanks! 2. Compute the and optimal strategies given the following: Pm=W1,W2,W3,W4 Pw1=m2,m3,m1,m4,ms Pm=W4,W2,W3,W1 P(w=m3,m1,m2,m4,m5 Pm3=W4,W3,W1,W2 Pw=m5,m4,mm2,m3 Pm4=W1,W4,W3,W2 Pw4=m1,m4,m5,m2,m3 Pms21 fevereiro 2025 -
 Answered: A wire has a current density of…21 fevereiro 2025
Answered: A wire has a current density of…21 fevereiro 2025 -
Solved Solving for a: a = (mm2) (m+m2+mp Equation 1021 fevereiro 2025
-
 freeshipping 0.5mm2-6mm2 M0 M1 M2 M3 Arabic Numerals M Type Clip21 fevereiro 2025
freeshipping 0.5mm2-6mm2 M0 M1 M2 M3 Arabic Numerals M Type Clip21 fevereiro 2025
você pode gostar
-
 VOCÊ CONHECE MESMO O JOGO FREE FIRE ?21 fevereiro 2025
VOCÊ CONHECE MESMO O JOGO FREE FIRE ?21 fevereiro 2025 -
 Extra time winner sees Spurs reach FA Cup quarter-finals21 fevereiro 2025
Extra time winner sees Spurs reach FA Cup quarter-finals21 fevereiro 2025 -
![8] Shiny Articuno! 2/4 Gen 1 shiny legendaries collected : r/ShinyPokemon](https://i.redd.it/hgtedepeddb61.jpg) 8] Shiny Articuno! 2/4 Gen 1 shiny legendaries collected : r/ShinyPokemon21 fevereiro 2025
8] Shiny Articuno! 2/4 Gen 1 shiny legendaries collected : r/ShinyPokemon21 fevereiro 2025 -
Our Father Prayer added a new photo. - Our Father Prayer21 fevereiro 2025
-
 Star Citizen21 fevereiro 2025
Star Citizen21 fevereiro 2025 -
 Empresário que perdeu aposta cobra jogador gaúcho por quebrar21 fevereiro 2025
Empresário que perdeu aposta cobra jogador gaúcho por quebrar21 fevereiro 2025 -
 Josuke Higashikata (JoJolion), Wiki21 fevereiro 2025
Josuke Higashikata (JoJolion), Wiki21 fevereiro 2025 -
 Aslan Voice - The Chronicles of Narnia: The Lion, the Witch and the Wardrobe (Movie) - Behind The Voice Actors21 fevereiro 2025
Aslan Voice - The Chronicles of Narnia: The Lion, the Witch and the Wardrobe (Movie) - Behind The Voice Actors21 fevereiro 2025 -
 How To Get Free Nanner Ringer Emote in Fortnite21 fevereiro 2025
How To Get Free Nanner Ringer Emote in Fortnite21 fevereiro 2025 -
 Como criar um caça-palavras: você nunca viu tão fácil!21 fevereiro 2025
Como criar um caça-palavras: você nunca viu tão fácil!21 fevereiro 2025